質問

ロジスティック回帰モデルを実行し、ロジット値の予測を行いました。これを用いてROC曲線上の点を求めました。

 from sklearn import metrics
 fpr, tpr, thresholds = metrics.roc_curve(Y_test,p)

私は知っている metrics.roc_auc_score はROC曲線の下での面積を与えます。最適なカットオフポイント（しきい値）を見つけるコマンドを教えてください。

どのように解決するのですか？

次のような方法があります。 を使って epi パッケージで しかし、私はPythonで同様のパッケージや例を見つけることができませんでした。

最適なカットオフポイントは、「真陽性率」が 高い で、「偽陽性率」が 低い . このロジックに基づき、最適な閾値を見つけるために、以下の例を引っ張ってきました。

Pythonのコードです。

import pandas as pd
import statsmodels.api as sm
import pylab as pl
import numpy as np
from sklearn.metrics import roc_curve, auc

# read the data in
df = pd.read_csv("http://www.ats.ucla.edu/stat/data/binary.csv")

# rename the 'rank' column because there is also a DataFrame method called 'rank'
df.columns = ["admit", "gre", "gpa", "prestige"]
# dummify rank
dummy_ranks = pd.get_dummies(df['prestige'], prefix='prestige')
# create a clean data frame for the regression
cols_to_keep = ['admit', 'gre', 'gpa']
data = df[cols_to_keep].join(dummy_ranks.iloc[:, 'prestige_2':])

# manually add the intercept
data['intercept'] = 1.0

train_cols = data.columns[1:]
# fit the model
result = sm.Logit(data['admit'], data[train_cols]).fit()
print result.summary()

# Add prediction to dataframe
data['pred'] = result.predict(data[train_cols])

fpr, tpr, thresholds =roc_curve(data['admit'], data['pred'])
roc_auc = auc(fpr, tpr)
print("Area under the ROC curve : %f" % roc_auc)

####################################
# The optimal cut off would be where tpr is high and fpr is low
# tpr - (1-fpr) is zero or near to zero is the optimal cut off point
####################################
i = np.arange(len(tpr)) # index for df
roc = pd.DataFrame({'fpr' : pd.Series(fpr, index=i),'tpr' : pd.Series(tpr, index = i), '1-fpr' : pd.Series(1-fpr, index = i), 'tf' : pd.Series(tpr - (1-fpr), index = i), 'thresholds' : pd.Series(thresholds, index = i)})
roc.iloc[(roc.tf-0).abs().argsort()[:1]]

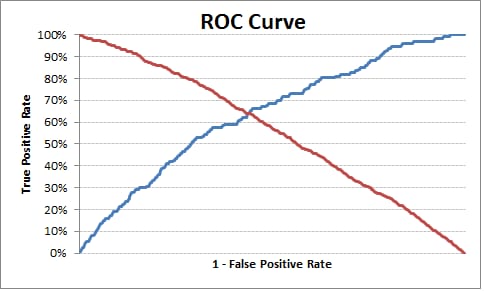
# Plot tpr vs 1-fpr
fig, ax = pl.subplots()
pl.plot(roc['tpr'])
pl.plot(roc['1-fpr'], color = 'red')
pl.xlabel('1-False Positive Rate')
pl.ylabel('True Positive Rate')
pl.title('Receiver operating characteristic')
ax.set_xticklabels([])

最適なカットオフポイントは0.317628で、これより上は1でなければ0とラベル付けできます。出力/チャートから、TPRが1-FPRを越えているところではTPRは63%、FPRは36%、TPR-（1-FPR）は現在の例では0に最も近いことがわかります。

出力します。

        1-fpr       fpr        tf     thresholds       tpr
  171  0.637363  0.362637  0.000433    0.317628     0.637795

これが役に立つことを願っています。

編集

単純化し、再利用性を高めるために、最適な確率のカットオフ点を求める関数を作りました。

Pythonのコードです。

def Find_Optimal_Cutoff(target, predicted):
    """ Find the optimal probability cutoff point for a classification model related to event rate
    Parameters
    ----------
    target : Matrix with dependent or target data, where rows are observations

    predicted : Matrix with predicted data, where rows are observations

    Returns
    -------     
    list type, with optimal cutoff value
        
    """
    fpr, tpr, threshold = roc_curve(target, predicted)
    i = np.arange(len(tpr)) 
    roc = pd.DataFrame({'tf' : pd.Series(tpr-(1-fpr), index=i), 'threshold' : pd.Series(threshold, index=i)})
    roc_t = roc.iloc[(roc.tf-0).abs().argsort()[:1]]

    return list(roc_t['threshold']) 


# Add prediction probability to dataframe
data['pred_proba'] = result.predict(data[train_cols])

# Find optimal probability threshold
threshold = Find_Optimal_Cutoff(data['admit'], data['pred_proba'])
print threshold
# [0.31762762459360921]

# Find prediction to the dataframe applying threshold
data['pred'] = data['pred_proba'].map(lambda x: 1 if x > threshold else 0)

# Print confusion Matrix
from sklearn.metrics import confusion_matrix
confusion_matrix(data['admit'], data['pred'])
# array([[175,  98],
#        [ 46,  81]])